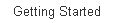
Sub-problem 1a: Maxwell Drive PM Peak Hour - Existing Conditions

Skipped Phase: What if skipped phases are ignored?
The next question is: do skipped phases make a difference? Indeed, they do. Exhibit 2-13 shows what happens if you don’t account for the fact that the phase is skipped. In the base case condition (Dataset 1), we’ve accounted for the skipped phases by using the 5+1.5+0.5 second timings we described earlier. In the Dataset 7, we’ve used the 10+3+1 second timings instead. In the case of Dataset 7, the delays for the westbound through-and-right are almost 3 times larger (42.7 seconds versus 16.7). This is because the cycle length is 5 seconds longer. That may be an unexpected result, but one that makes sense. The v/c ratio started out at 0.87 with the 5.0+1.5+0.5 timings and it becomes 1.01 when the 10+3+1 timings are used. That change in v/c ratio will produce a dramatic result. The change is arguably dramatized because the signal timings for the other phases haven’t been adjusted to reflect the change in the phase 2 timings. If we do that, and strive to get a balance in v/c ratios instead of delays, we can obtain the results presented in Dataset 8. The v/c ratios for the critical movements are nearly balanced at 0.75, 0.66, and 0.75, and the westbound delays are now lower than in the base case at 15.2 sec/veh. However, you should note that the delays for all the other movements are higher. The delay for the eastbound left is now 35.5 sec/veh instead of 18.8; for the eastbound through, it’s 6.9 instead of 5.3; and for the southbound approach, it is now 32.9 and 42.2 sec/veh instead of 17.3 and 20.8 sec/veh respectively for the left and right. The table below includes eastbound arrival type 2 and includes heavy vehicle considerations.

These three solutions also illustrate the huge variations in delay that can be achieved depending on the signal timings you use. It’s important to tell your client and the other stakeholders what signal timing philosophy you’re using so they know, or have some idea, what results to expect. Ideally, you’re using a philosophy that reflects what the signal will do in the field, but since that is heavily influenced by timing parameters employed (minimum greens, maximum greens, gap times and a host of other values), it’s hard to exactly duplicate the field performance.

As an alternative to this observational approach, you might also consider applying one of the signal timing estimation procedures described in Chapter 16, Appendix B of the HCM. The decision on which approach is most appropriate depends on the available data, the required accuracy, and available time and resources.